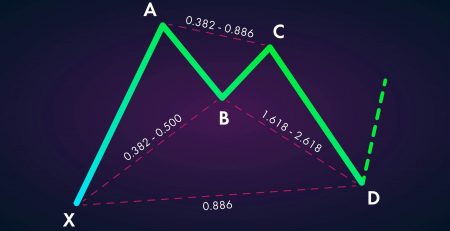
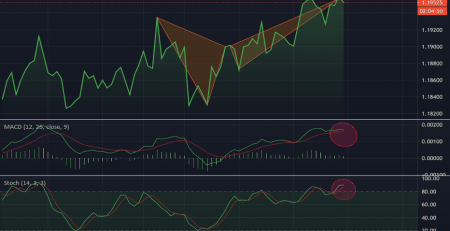
Fibonacci ratios

Fibonacci numbers are also mentioned in any discussion of harmonic patterns, as this pattern uses Fibonacci ratios. The Fibonacci number series was first introduced by Leonardo Fibonacci, the great Italian and European mathematician of the thirteenth century. On his return from a trip to Egypt, he introduced this series of numbers in his book, The Account Book. Fibonacci ratios, especially the “Golden Ratio” ratios, can be found in nature, such as the arrangement of snowflakes or the number and placement of sunflower seeds, and have been observed in human organs such as DNA spirals.

The Fibonacci number series is defined in such a way that, apart from the first two numbers, the next numbers are obtained from the sum of their two previous numbers. The numbers in this series are:

…, 1,1,2,3,5,8,8,13,21,34,55,589,144,233

The main reason for the popularity of this sequence of numbers is that the result of dividing each sequence number by its predecessor is the golden number of 1.618, also known as the “golden ratio” or “fi number”. This is the order of the numbers that Fibonacci emphasized many years ago, and the relationship between these numbers (the golden ratio) in financial markets and the stock market is also very useful for analysts.

There are famous sayings in technical analysis that say everything is summed up in price, including human behavior. As has been said, Fibonacci proportions are present in all human beings, and man in his subconscious, without knowing it, tends to these proportions and numbers and sees them as beautiful, as he makes them very eye-catching in paintings and architectures that use these proportions. .

These Fibonacci ratios also affect people’s behavior in stock markets and their transactions. As a result, these ratios can be used to predict prices, market entry and exit points, identify support and resistance levels, and even time forecasts. Fibonacci ratios are invisible levels of support and resistance that are determined for the trader before the market reaches them and even without a price history.

The most common Fibonacci ratios are 0.382, 0.50, 0.618, 0.786 and 1/00, 1/272, / 1/618. There are other ratios such as 0.236, 2.618 and 4.236 that do not apply as much as the mentioned ratios, however, the most important Fibonacci ratios can be considered 0.382, 0.618 and 1.618.

How can the Fibonacci levels of correction [1] and expansion [2] be calculated through the above progression?

61.8% = Divide each escalation sentence by the next sentence (after the thirteenth sentence).
For example: (approximately) 55/18 = 0.618

• 161.8% = Divide each collision sentence by the previous sentence.

• For example: 89/55 = 1,618 ٫ which is called the “golden ratio”.
• 38.2% = Divide each sentence by the second sentence after it
  For example: 55/144 = 0.382)
• 23.6% = Divide each sentence by the third sentence after it
  For example: (approximately) 34/144 = 0.236

Ratios of 0%, 50%, 100% and 200% are not Fibonacci numbers, but they are still used by some traders.

The most important levels of Fibonacci in technical analysis

161.8%
61.8%
38.2%
What makes Fibonacci analysis so popular?

Fibonacci levels are geometric numbers, so extension and correction levels look eye-catching after drawing.
Fibonacci levels provide tangible reference points, thus preventing the subject from being abstracted if used properly.
Fibonacci expansion and correction levels are invisible levels of support and resistance.

What is the difference between Fibonacci extension and retracement levels?

Fibonacci retracement levels represent levels below 100% of a price wave, while expansion levels also represent levels above 100%. Fibonacci levels are used as support and resistance levels, these levels can also be used as a tool to determine the price target.